Direct Variation Linear Relations Partial Variation Proportional Reasoning Systems of Linear Equations

Direct and Partial Variation Linear Relations

$Tech Weigh In - iPad 2 Weigh In 3 Act Math Task - What's the Q$

In the Ontario grade 9 applied and academic math courses, students do a lot of work with direct (proportional) and partial variation linear relations. This task was created to assist in adding context to these types of relations and help students build an understanding of both by using intuition, logic, and prior knowledge.

Special thanks to Justin Levack for coming up with the idea and pushing me to actually record this with him when I was running out of steam at the end of the school year!

Task #1 – iPad 2 Weigh In

Act 1 – Introduce the Problem

Show this video:

What’s the question?

Get your students talking about what they think this math task is going to focus on. Writing the questions out is always helpful.

Here are just a few of the questions that came up today when we used this task for the first time:

How much does 3 iPads weigh?
How much does 1 iPad weigh?
How many scales would weigh the same as the stack of iPads on the table?
What is the cost per gram/ounce/kg/etc.?

All great questions that should be recognized! While we could try to answer as many questions as possible, today our focus was on the weight of 1 iPad.

Time to make some predictions!

Act 2 – Giving Some Information

After students are happy with their predictions, start asking students what information they want. In this case, I have pretty specific information, but my students can usually come up with something similar that could help us out.

Show them this video:

$Tech Weigh In - iPhone 2 Weigh In 3 Act Math Task$

Now we can set the students free to work out the problem. At the beginning of the semester, my grade 9 applied students might struggle to get started due to a low level of confidence in math, but today they were done in a matter of 20 seconds or so. They’ve come a real long way!

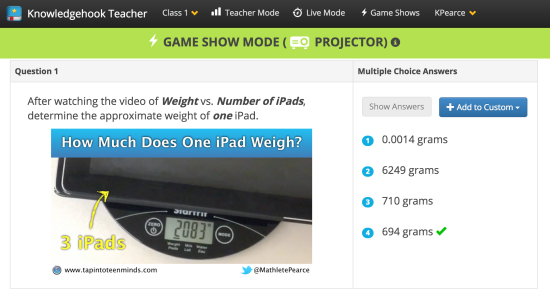
I also created a Custom Gameshow in Knowledgehook to allow me to get some instant feedback as students worked. The Gameshow Tool now has a feature that allows students to upload their work and then I can display student work samples to consolidate thinking. It was pretty rad!

$Tech Weigh In 3 Act Math Task - Knowledgehook Gameshow Question$

You can grab a PDF copy of the Custom Gameshow here.

Act 3 – Watch the Solution

iPad 2 Weigh In Extensions

Although this is a simple proportion, I try to get students to see the other connections that can be made to linear relations including direct variation. Some additional extension questions could include:

Is this relationship an example of a direct or partial variation? How do you know?
Find the initial value and rate of change.
Determine an equation that relates the weight and number of iPad 2s
How Many iPads would weigh 27,066 grams?

Task #2 – MacBook Pro & iPad Minis Weigh In

Since I do these problems back-to-back, students already have a feel for what we’ll likely be exploring. You could try to pull out some more question ideas, but I usually find that the students are already hooked in and are actually more interested in getting to the math. I do, however, try to get some predictions out there just to ensure that they have some way to judge how realistic their answers are.

Act 2 – Giving Some Information

Show them this video:

The question is: How much does the MacBook Pro weigh in at?

$MacBook Pro and iPad Mini Weigh In 3 Act Math Task - Act 2$

Again, students are now ready to be set free to solve the problem. There is no pre-teaching necessary here. Most students will intuitively be able to determine the weight of 1 iPad Mini and ultimately use the formula for slope of a line by accident as they usually do in Thick Stacks. Let them do it on their own and consolidate later.

Surprisingly, one student even wrote out their solution as a system of linear equations and used elimination to eliminate the MacBook Pro completely:

I found this to be fascinating because I had never even considered this as a potential problem to introduce the concept of elimination to a group of students working with systems. Not my intent at all with this problem, but you bet I’ll be using it in that case from now on!

Act 3 – Watch the Solution

MacBook Pro and iPad Minis Weigh In Extensions

Here are some extension questions to complement this partial variation relation:

Is this relationship an example of a direct or partial variation? How do you know?
Find the initial value and rate of change.
Determine an equation that relates the Weight and Number of iPad Minis
Determine the approximate number of iPad Minis it would take for the entire stack (including the MacBook) to weigh 29,005 grams?

Access Resources

Check out all of the resources here. Or, you can grab what you want individually below:

Task #1 – iPad 2 Weigh In – Act 1 [VIDEO]
Task #1 – iPad 2 Weigh In – Act 2 [VIDEO]
Task #1 – iPad 2 Weigh In – Act 3 [VIDEO]
Task #2 – MacBook Pro and iPad Mini Weigh In – Act 2 [VIDEO]
Task #2 – MacBook Pro and iPad Mini Weigh In – Act 3.1 [IMAGE]
Task #2 – MacBook Pro and iPad Mini Weigh In – Act 3.2 [VIDEO]
Slide Deck for Both Tasks [KEYNOTE]

Student Exemplars

I only managed to grab one photo of student work today (ooops!) so please send your student work my way or add the images in the comments (yes, you can add images!) so others can learn from your students!

Have you tried the task? Let us know how to make it better in the comments!

New to Using 3 Act Math Tasks?

Download the 2-page printable 3 Act Math Tip Sheet to ensure that you have the best start to your journey using 3 Act math Tasks to spark curiosity and fuel sense making in your math classroom!

DOWNLOAD TIP SHEET

Share With Your Learning Community:

About Kyle Pearce

I’m Kyle Pearce and I am a former high school math teacher. I’m now the K-12 Mathematics Consultant with the Greater Essex County District School Board, where I uncover creative ways to spark curiosity and fuel sense making in mathematics. Read more.

Access Other Real World Math Tasks

← Graphing Stories - 3 Act Math Style! Camera Case & Pads of Paper Weigh In →

Search More 3 Act Math Tasks

Algebra [A-CED.1, A-CED.2, A-REI.6]

Functions [F-BF.1, F-BF.3, F-IF.4, F-LE.1, F-LE.2, F-LE.3, F-TF.5]

Geometry [G-C.5, G-C.8, G-C.9, G-GMD.3, G-GMD.4, G-GPE.4, G-GPE.5, G-GPE.7, G-MG.1, G-MG.2, G-SRT.11]

Grade 1 [1.NBT.4, 1.OA.1, 1.OA.6, 1.OA.A.1, 1.OA.B.3, 1.OA.B.4, 1.OA.C.5, 1.OA.C.6]

Grade 2 [2.NBT.5, 2.NBT.B.5, 2.NBT.B.8, 2.NBT.B.9, 2.OA.2, 2.OA.A.1, 2.OA.B.2]

Grade 3 [3.MD.C.5, 3.NBT.2, 3.NF.1, 3.NF.2, 3.NF.3, 3.NF.A.1, 3.OA.1, 3.OA.5, 3.OA.9]

Grade 4 [4-MD.3, 4.MD.1, 4.MD.2, 4.NBT.6, 4.NF.3, 4.NF.5, 4.NF.6, 4.OA.1, 4.OA.5]

Grade 5 [5.B1.7, 5.D1.3, 5.D1.6, 5.MD.1, 5.MD.3, 5.MD.4, 5.MD.5, 5.NBT.2, 5.NBT.3, 5.NBT.6, 5.NBT.7, 5.NF.1, 5.NF.2, 5.NF.3, 5.NF.4, 5.NF.5, 5.OA.1, 5.OA.2, 5.OA.3]

Grade 6 [6.EE.1, 6.EE.2, 6.EE.5, 6.EE.6, 6.EE.7, 6.G.1, 6.G.2, 6.NS.1, 6.NS.3, 6.NS.6, 6.NS.B.3, 6.NS.C.6, 6.NS.C.7, 6.NS.C.8, 6.RP.1, 6.RP.2, 6.RP.3, 6.RP.A.1, 6.RP.A.2, 6.RP.A.3, 6.RP.A.3.C]

Grade 7 [7.EE.3, 7.EE.4, 7.EE.A.1, 7.G.3, 7.G.4, 7.G.6, 7.NS.A.1, 7.NS.A.2, 7.RP.1, 7.RP.3, 7.RP.A.2.B, 7.RP.A.3, 7.SP.2, 7.SP.5, 7.SP.6]

Grade 8 [8.EE.1, 8.EE.5, 8.EE.6, 8.EE.7, 8.EE.8, 8.F.2, 8.F.3, 8.F.4, 8.F.5, 8.G.5, 8.G.6, 8.G.7, 8.G.9, 8.SP.1]

Grade 9 Kindergarten [K.CC.A.1, K.NBT.A.1, K.OA.A.1, K.OA.A.2, K.OA.A.3]

Practice [MP.1, MP.2, MP.3, MP.4, MP.6, MP.7]

Statistics & Probability [S-ID.6, S-MD.4]

Andrew Stadel Brennan Jones Brian Marks Cathy Yenca Dan Meyer Graham Fletcher Jim Pardun John Scammell Jon Orr Justin Levack Kyle Pearce Mike Wiernicki Mishaal Surti Nathan Kraft Robert Kaplinsky Wendy Goodman

7 Comments

MaryAnn Moore on June 4, 2015 at 11:36 pm

Great stuff! Adding this one to my virtual file cabinet. What writing implement did your student use to write on his/her desk? Does it erase off cleanly? That looks pretty cool.
Reply
- Kyle Pearce on June 13, 2015 at 2:03 pm
  
  Thanks for the feedback! My students use dry-erase markers or dry-erase crayons. I prefer the crayons for environmental reasons (no plastic going into the landfill), but they are a bit more of a hassle to wipe off. You definitely need a spray bottle and rag vs. just a dry-eraser. Students seem to like writing on the desks and I can see that they are less worried about making a mistake when it looks ‘messy’ 🙂
  Reply
Jeff Sellers on August 13, 2016 at 11:26 am

Really like this task! Question—you mention direct/partial variation, initial value/rate of change. Here’s where I get stuck with using 3-act tasks: how much teaching have you done with initial value/rate of change or direct/partial variation? And what does that part look like? Through attending conferences & reading various books, I’ve been shamed into “worksheets practice = bad practice”. So I’m trying to get away from them. I have full inclusion classes and I just need to find a way for students to make a connection between the 3-Act task and what they will see on their State test (I know it shouldn’t be about the test, but when my evaluation is tied to it, it kind of matters to me). Thanks for any advice. I feel like I see all of these great 3-Act tasks, but I can never seem to get a straight answer of how to build up or build upon the 3-Act task. Again, thanks for any help you can provide!
Reply
- Kyle Pearce on August 17, 2016 at 10:10 pm
  
  Hi Jeff,
  
  Thanks for stopping by and for the great question. I’d agree with you that many are implicit (or explicit) about their feelings about worksheets. I’m definitely guilty of it and have been really trying to reel that back. It is less about whether you use a worksheet and more about what the experience and culture of your classroom is like. I’m sort of past the worrying about what other people think regarding worksheets as they do serve a purpose assuming the activity / practice is worthwhile. In regards to the pre-teaching, etc. I am big on inquiry in order to create a need for the math. 3 Act Tasks are great to hook students in and then, I try to ensure that there is a way for students to use prior knowledge to solve the problem. Any new content we are trying to introduce should be related somehow to prior knowledge of the student. Once students solve it in some way, we consolidate the learning to the new, usually more efficient approach to add that to their tool belt. So, in regards to this task in particular, I might use it at a different point than you would. It really comes down to how YOU want to go about it and where you see it best fitting for your group. I definitely don’t want to preload too much info prior to doing a question or all the fun/curiosity is sucked out of the problem.
  Not sure if I’ve hit your question as well as you’d like, but I hope it gives you something to think about in these last few weeks of summer! Good luck and stay in touch!
  Reply
  - Jeff Sellers on August 18, 2016 at 9:10 am
    
    Thanks for the info! It is a big help to see the 3-Act as a hook and also as a method for students to find their way to the answer, but afterwards, show them the easier path. I appreciate the feedback!
    Reply
    - Kyle Pearce on August 19, 2016 at 9:47 pm
      
      Keep in touch and let me know how things go!
      Reply
  - Jeff Sellers on August 18, 2016 at 9:10 am
    
    Thanks for the info! It is a big help to see the 3-Act as a hook and also as a method for students to find their way to the answer, but afterwards, show them the easier path. I appreciate the feedback!
    Reply