Using Context to Introduce Parallel, Perpendicular or Neither

This 3 act math task was created using video clips from the Walk Out 3 Act Math Task as a way to get students wondering about parallel and perpendicular lines. Here’s the expectation from the grade 9 academic course in Ontario:

• AG2.04 – identify, through investigation, properties of the slopes of lines and line segments (e.g., direction, positive or negative rate of change, steepness, parallelism, perpendicularity), using graphing technology to facilitate investigations, where appropriate.

After spending some time asking folks on Twitter about how they introduce the concept of parallel and perpendicular lines, I found that we were all doing something pretty similar.

To investigate parallel lines:

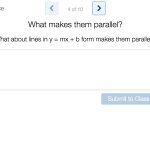
• Give students two lines with the same slope in slope/y-intercept form;
• ask them to graph the lines; and,
• ask them what they notice.

The process was pretty similar for perpendicular lines:

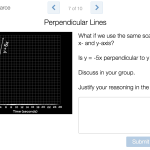
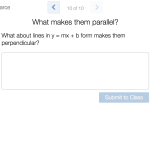
• Give students two lines with negative reciprocal slopes in slope/y-intercept form;
• ask them to graph the lines; and,
• ask them what they notice.

After students complete the two investigations, a consolidation would take place. I’ve been doing something similar to this for about 10 years and while it does appropriately address the parallel and perpendicular portions of the expectation through investigation, it felt more like I spoon fed the investigation a bit too much.

While it is pretty easy to find a contextual situation where comparing two parallel lines makes logical sense, I really struggled to find a useful case for comparing two perpendicular lines. You can compare the linear relations representing the cost of two different taxi companies as parallel lines, but it makes absolutely no sense if you try to make a case where the linear relations are perpendicular.

When distance-time graphs came up in my course, I created the Walk Out tasks and finally found a way to at least spark the conversation about parallel and perpendicular lines.

Here it is:

Act 1 – Introduce the Problem

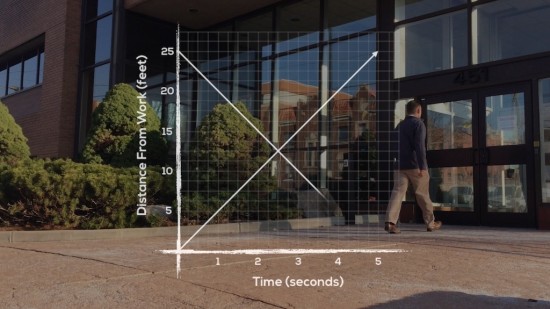
Show this video.

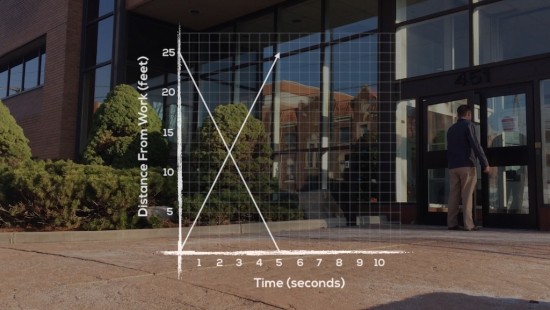
If you have already used the Walk Out 3 Act Math Task with your class, you could probably just show this image:

Ask students what they wonder? What questions come to mind?

Write down some of the questions/ideas that students share.

Hopefully one or more students come up with something like “are they perpendicular or not?”

Once sharing out those questions that come up as a group, I ask them:

Are the lines parallel, perpendicular, or neither? How do you know?

I give them a minute to discuss with their groups.

In grade 9 academic, it might be a good idea to ask the group what it means for two lines to be parallel or perpendicular. What does it look like? What are some examples? This is the first time those concepts come up in the math curriculum in Ontario, so I never assume everyone has an understanding.

Teacher Move: It should be noted that my intention with the video/image is that the lines do appear to be perpendicular, but the scale on the x- and y-axis’ are not equivalent. This gives the viewer the impression that the lines are parallel, but as you’ll see in the next few videos/images, they are not.

Act 2 – Give Some Information

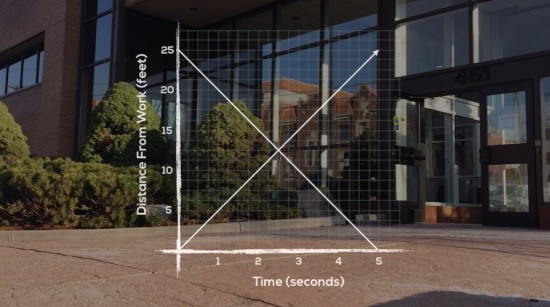
After allowing students to argue it out a bit, I play this video.

Alternatively, here’s the image:

Then, I show them this video to give a bit more information.

Give students more time to discuss and share out. I usually ask students to share out who feels confident in their original guess and who wants to update their prediction.

Act 3 – Show The Answer

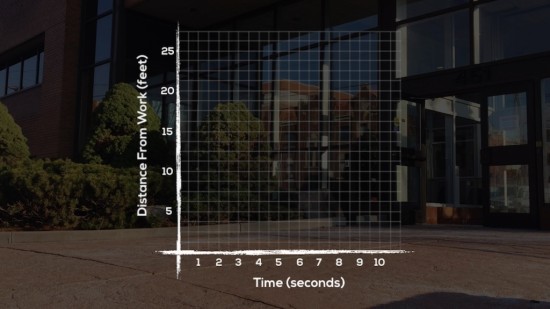
Now, I play this video.

Alternatively, here’s the image:

Here are a couple more videos you might want to show as they change the scale to 1:1, but aren’t necessary:

Act 3, Scene 2
Act 3, Scene 3

Here’s some screenshots from the Desmos Parallel and Perpendicular Lines Activity Builder Activity:

Task Resources:

Here’s all the links in one convenient spot for this activity to go off without a hitch:

Act 1 [video]
Act 2 [scene 1 video | scene 2 video]
Act 3 [scene 1 video | scene 2 video (optional) | scene 3 video (optional)]
YouTube Playlist [All Videos]
Desmos Activity Builder [Try It | Copy It]
Knowledgehook Custom Gameshow [Try It | Copy It]

Make sure to leave a comment if you try out the task! Would love to get some tips on how to make this better.

New to Using 3 Act Math Tasks?

Download the 2-page printable 3 Act Math Tip Sheet to ensure that you have the best start to your journey using 3 Act math Tasks to spark curiosity and fuel sense making in your math classroom!

DOWNLOAD TIP SHEET

About Kyle Pearce

I’m Kyle Pearce and I am a former high school math teacher. I’m now the K-12 Mathematics Consultant with the Greater Essex County District School Board, where I uncover creative ways to spark curiosity and fuel sense making in mathematics. Read more.

• Follow
• Follow
• Follow
• Follow
• Follow
• Follow

Access Other Real World Math Tasks

Search More 3 Act Math Tasks