Ontario Alignment By Overall Expectation
So earlier this month, I was in Sudbury, Ontario delivering an Apple Professional Development session for the Sudbury Catholic District School Board. I had always heard about the “Big Nickel” monument and thought I should search it out. On my way, Justin Levack sent me a text saying I should see what kind of 3 Act Math Task I could think of. This time, rather than trying to develop a question on my own, I tossed it out to the Twitterverse:
Thoughts on what to do with this one? @ddmeyer @mathycathy @robertkaplinsky @mr_stadel @Ryan7Read #maths #mathchat pic.twitter.com/qQlh8oTW3p
— Kyle Pearce (@MathletePearce) September 19, 2014
As expected, I had some ideas coming to me within minutes including these:
@MathletePearce @ddmeyer Get the roasted red pepper soup, whatever you do! And..how many nickels would have to be rendered to make THIS one?
— Cheryl Geoghegan (@mathsuds) September 19, 2014
@MathletePearce If that was made of nickel how much would it be worth? How many nickels?
— Dan Meyer (@ddmeyer) September 19, 2014
@mr_stadel My favorite question so far, Andrew! No rest for me until I know the answer @MathletePearce @ddmeyer @robertkaplinsky @Ryan7Read
— Cathy Yenca (@mathycathy) September 20, 2014
So, just like that, Cathy Yenca went and solved Dan Meyer and Andrew Stadel‘s question and was kind enough to share her work with us so we can all use this task when a related learning goal comes up.
Act 1: Introducing the Problem
Show your students this video or the photo below:
Act 2: Students Request Information and You Give It To Them
The approach you choose to have your students answer whether constructing the Big Nickel with actual Canadian Nickels vs. using the material they actually used to build the monument would be more cost effective will depend on the level of your mathematics students. Here, I’ll give two approaches to make this task accessible by more students.
All students would benefit from these two photos:
Diameter of the Big Nickel in Sudbury, Ontario

Big Nickel Divided into 12 Triangles

Thickness of the Big Nickel
The thickness of the Big Nickel will serve as the height of the prism in order to calculate the volume. By taking the area of the base and multiplying by the thickness (height), students will know how much space the Big Nickel occupies:
Thickness of a 1951 Canadian Nickel
The thickness of a 1951 Canadian Nickel will serve as the “height” in the volume formula when calculating how much space is occupied by an actual nickel:
Method #1: No Trigonometry – Volume and Proportional Reasoning
If your students have not covered trigonometry or primary trigonometric ratios yet, you’ll want to give the students a little more information. Here are some details you can give your students:
Base and Height of One (1) Triangular Section of Big Nickel
Students can use these dimensions to determine the area of one triangular section of Big Nickel, then multiply by 12 to get the area of the base in order to help them find the total volume:
Base and Height of One (1) Triangular Section of a 1951 Canadian Nickel
Students can use these dimensions to determine the area of one triangular section of a 1951 Canadian Nickel as they work towards finding the volume:
More Info Provided: Width of Actual Nickel
Thanks to John Golden for sharing the following image on Twitter. The online math community is “Golden”!

Method #2: Using Primary Trigonometric Ratios
If your students have covered the primary trigonometric ratios, you can opt to use the following images that force students to use trig ratios to find a working solution:
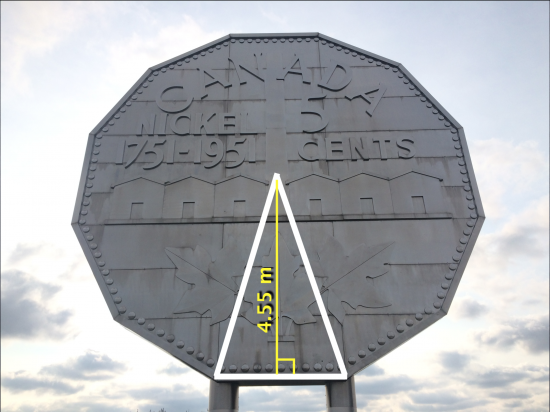
Height of a Right Angle Triangle in Big Nickel
Students know that the diameter of Big Nickel is 9.1 m and thus the height of the right angle triangle we can form in one of the 12 triangular sections
is 4.55 m. From here, students must use their knowledge of primary trigonometric ratios to find the base of the triangular section:
Using a similar approach to the above image, students must also use their knowledge of primary trigonometric ratios to find the base of a triangular section of a 1951 Canadian Nickel shown below:
Act 3: Students Compare Their Cost to Actual Cost
Once students determine the volume of Big Nickel and a 1951 Canadian Nickel, they can determine how many nickels it would take to build the monument as well as the total cost to do so.
The Big Nickel was completed in 1964 for approximately $35,000 according to Wikipedia. Information about the 1951 Canadian Nickel also according to Wikipedia.
My assumption is that the nickels would be melted down to create the monument, but some students may assume you’re just “dropping the nickels in” or something along those lines. Great discussion to be had there.
Sequels, Extensions & Additional Images
Here are some additional extensions and images that are included in the slide deck available for download:
- The City of Windsor wants to build a “Double Big Nickel” where the total volume is doubled. Can you just double the dimensions?
- The WFCU Arena in Windsor cost a total of $71 Million to build. What percentage of that cost would it take to construct the Big Nickel?
- When constructing the Big Nickel, construction workers need to know the interior angle measures in order to build it. What are they?
- If the Big Nickel needs to be taken off-side for repair, what would the interior angles be for the supports needed?
- If resurfacing the entire Big Nickel, how much would it cost?
Knowledgehook Embedded Gameshow Formative Assessment
Check out this nifty new feature that Knowledgehook came up with called “Gameshow Embedding”. Not only can you create a Gameshow as a formative assessment, you can actually embed it as a full Gameshow in your blog/website/LMS or embed each question individually throughout your post! Here’s a couple sample problems I created for this task:
Check Out @MathyCathy’s Thoughts & Working Solution
Cathy Yenca was the first to answer Dan Meyer and Andrew Stadel’s question(s) and she was kind enough to share her work via a TACKK! Click here or on the image below to see the full solution:
Cathy also blogged about our co-creation of this task from a great distance over a very short period of time! Read about it here!
Direct Links to Resources Available For Download:
- Act 1 [VIDEO]
- Slide Deck Images [JPG FILES]
- Slide Deck Presentation [KEYNOTE FILE]
Did you try it?
Be sure to share your experience in the comments down below…
New to Using 3 Act Math Tasks?
Download the 2-page printable 3 Act Math Tip Sheet to ensure that you have the best start to your journey using 3 Act math Tasks to spark curiosity and fuel sense making in your math classroom!
Share With Your Learning Community:

About Kyle Pearce
I’m Kyle Pearce and I am a former high school math teacher. I’m now the K-12 Mathematics Consultant with the Greater Essex County District School Board, where I uncover creative ways to spark curiosity and fuel sense making in mathematics. Read more.
Access Other Real World Math Tasks
Search More 3 Act Math Tasks
Grade 2 [2.B1.1, 2.B1.3, 2.B2.1, 2.B2.2, 2.B2.3, 2.B2.4, Measurement - M1, Number Sense and Numeration - NS1, Number Sense and Numeration - NS2, Number Sense and Numeration - NS3]
Grade 3 [3.B1.5, 3.B2.1, 3.B2.3, 3.B2.7, Measurement - M1, Number Sense and Numeration - NS1, Number Sense and Numeration - NS3]
Grade 4 [4.B2.1, 4.B2.4, 4.E2.5, 4.E2.6, Measurement - M1, Number Sense and Numeration - NS1, Number Sense and Numeration - NS3, Patterning and Algebra - PA2]
Grade 5 [5.B1.7, 5.B2.9, 5.D1.3, 5.D1.6, 5.E2.6, 5.F1.2, 5.F1.5, Measurement - M1, Measurement - M2, Number Sense and Numeration - NS1, Number Sense and Numeration - NS3, Patterning and Algebra - PA2]
Grade 6 [6.B2.12, 6.B2.9, Data Management and Probability - DP3, Measurement - M1, Measurement - M2, Number Sense and Numeration - NS1, Number Sense and Numeration - NS2, Number Sense and Numeration - NS3, Patterning and Algebra - PA1, Patterning and Algebra - PA2]
Grade 7 [7.B1.3, 7.B1.4, 7.B1.7, 7.B2.2, 7.B2.3, 7.C1.1, 7.C1.2, 7.C1.3, 7.C1.4, 7.D1.6, Data Management and Probability - DP3, Geometry and Spatial Sense - GS1, Measurement - M1, Measurement - M2, Number Sense and Numeration - NS1, Number Sense and Numeration - NS2, Number Sense and Numeration - NS3, Patterning and Algebra - PA1, Patterning and Algebra - PA2]
Grade 8 [8.B1.4, 8.B2.5, 8.C1.1, 8.C1.2, 8.C1.3, 8.C1.4, Data Management and Probability - DP1, Data Management and Probability - DP3, Geometry and Spatial Sense - GS2, Measurement - M1, Measurement - M2, Number Sense and Numeration - NS1, Number Sense and Numeration - NS2, Number Sense and Numeration - NS3, Patterning and Algebra - PA1, Patterning and Algebra - PA2]
Grade 9 [9.B3.5, 9.C3.1, 9.C3.2, 9.C3.3]
Kindergarten [k.15.1, k.15.10, k.15.2]
MAP4C [Mathematical Models - MM1, Mathematical Models - MM2, Mathematical Models - MM3]
MAT1LMAT2LMBF3C [Data Management - DM1, Data Management - DM2, Geometry and Trigonometry - GT1, Geometry and Trigonometry - GT2, Mathematical Models - MM1, Mathematical Models - MM2, Mathematical Models - MM3]
MCF3M [Exponential Functions - EF2, Quadratic Functions - QF1, Quadratic Functions - QF2, Quadratic Functions - QF3, Trigonometric Functions - TF1, Trigonometric Functions - TF3]
MCR3U [Characteristics of Functions - CF1, Characteristics of Functions - CF2, Exponential Functions - EF2, Exponential Functions - EF3, Trigonometric Functions - TF3]
MCT4C [Exponential Functions - EF1, Trigonometric Functions - TF3]
MCV4U [Derivatives and Their Applications - DA2]
MDM4U [Counting and Probability - CP2, Organization of Data For Analysis - DA2, Probability Distributions - PD1, Statistical Analysis - SA1, Statistical Analysis - SA2]
MEL4EMFM1P [Linear Relations - LR1, Linear Relations - LR2, Linear Relations - LR3, Linear Relations - LR4, Measurement and Geometry - MG1, Measurement and Geometry - MG2, Measurement and Geometry - MG3, Number Sense and Algebra - NA1, Number Sense and Algebra - NA2]
MFM2P [Measurement and Trigonometry - MT1, Measurement and Trigonometry - MT2, Measurement and Trigonometry - MT3, Modelling Linear Relations - LR1, Modelling Linear Relations - LR2, Modelling Linear Relations - LR3, Quadratic Relations in y = ax^2 + bx + c Form - QR1, Quadratic Relations in y = ax^2 + bx + c Form - QR2, Quadratic Relations in y = ax^2 + bx + c Form - QR3]
MHF4U [Characteristics of Functions - CF3, Exponential and Logarithmic Functions - EL2, Exponential and Logarithmic Functions - EL3]
MPM1D [AG3, Analytic Geometry - AG1, Analytic Geometry - AG2, LR1, LR2, LR3, MG1, MG2, MG3, NA1, Number Sense and Algebra - NA2]
MPM2D [AG1, AG2, AG3, QR2, Quadratic Relations - QR3, Quadratic Relations - QR4, T2, T3]
Functions [F-BF.1, F-BF.3, F-IF.4, F-LE.1, F-LE.2, F-LE.3, F-TF.5]
Geometry [G-C.5, G-C.8, G-C.9, G-GMD.3, G-GMD.4, G-GPE.4, G-GPE.5, G-GPE.7, G-MG.1, G-MG.2, G-SRT.11]
Grade 1 [1.NBT.4, 1.OA.1, 1.OA.6, 1.OA.A.1, 1.OA.B.3, 1.OA.B.4, 1.OA.C.5, 1.OA.C.6]
Grade 2 [2.NBT.5, 2.NBT.B.5, 2.NBT.B.8, 2.NBT.B.9, 2.OA.2, 2.OA.A.1, 2.OA.B.2]
Grade 3 [3.MD.C.5, 3.NBT.2, 3.NF.1, 3.NF.2, 3.NF.3, 3.NF.A.1, 3.OA.1, 3.OA.5, 3.OA.9]
Grade 4 [4-MD.3, 4.MD.1, 4.MD.2, 4.NBT.6, 4.NF.3, 4.NF.5, 4.NF.6, 4.OA.1, 4.OA.5]
Grade 5 [5.B1.7, 5.D1.3, 5.D1.6, 5.MD.1, 5.MD.3, 5.MD.4, 5.MD.5, 5.NBT.2, 5.NBT.3, 5.NBT.6, 5.NBT.7, 5.NF.1, 5.NF.2, 5.NF.3, 5.NF.4, 5.NF.5, 5.OA.1, 5.OA.2, 5.OA.3]
Grade 6 [6.EE.1, 6.EE.2, 6.EE.5, 6.EE.6, 6.EE.7, 6.G.1, 6.G.2, 6.NS.1, 6.NS.3, 6.NS.6, 6.NS.B.3, 6.NS.C.6, 6.NS.C.7, 6.NS.C.8, 6.RP.1, 6.RP.2, 6.RP.3, 6.RP.A.1, 6.RP.A.2, 6.RP.A.3, 6.RP.A.3.C]
Grade 7 [7.EE.3, 7.EE.4, 7.EE.A.1, 7.G.3, 7.G.4, 7.G.6, 7.NS.A.1, 7.NS.A.2, 7.RP.1, 7.RP.3, 7.RP.A.2.B, 7.RP.A.3, 7.SP.2, 7.SP.5, 7.SP.6]
Grade 8 [8.EE.1, 8.EE.5, 8.EE.6, 8.EE.7, 8.EE.8, 8.F.2, 8.F.3, 8.F.4, 8.F.5, 8.G.5, 8.G.6, 8.G.7, 8.G.9, 8.SP.1]
Grade 9Kindergarten [K.CC.A.1, K.NBT.A.1, K.OA.A.1, K.OA.A.2, K.OA.A.3]
Practice [MP.1, MP.2, MP.3, MP.4, MP.6, MP.7]
Statistics & Probability [S-ID.6, S-MD.4]


















I teach adapted math applications. We stopped at area of the nickel. It was a lot of fun and a great application of triangles, which we study a lot in our classes.
So awesome to hear! Thanks for sharing your experience with us!