York 4 Mathematics Association Math Professional Development Workshop

May 2017

Thanks for inviting me to learn with the York 4 Mathematics Association Chapter of OAME for an evening of math professional development! The group was energetic and excited to learn about meaningful manipulative use, sparking curiosity and building conceptual understanding across the grades.

Here’s a summary of what we explored today. Looking forward to connecting again soon!

---

After a brief presentation about avoiding the rush to the algorithm, we took some time to explore a 3 act math task with a very low-floor and high-ceiling, called Toilet Paper Rolls. The resources are not yet posted on the web, but I hope to do this soon!

---

Counting Principles

We briefly discussed the importance of counting and quantity principles, but focused on the importance of unitizing. A full summary is below:

[postshortcode the_query=”p=18603″]

---

Exploring the Progression of Multiplication

We made a leap from counting principles and unitizing to multiplication and specifically, using arrays and area models.

[postshortcode the_query=”p=17501″]

As an aside, you might consider looking at the “Japanese Multiplication” or “Stick Multiplication” which is derived from base ten block representation:

[postshortcode the_query=”p=18762″]

---

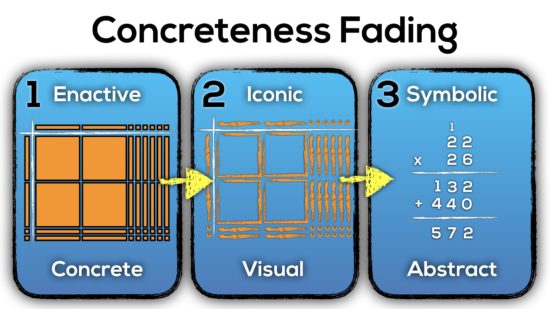
Concreteness Fading

We attempted to summarize the use of manipulatives on a continuum called “Concreteness Fading”. While the name suggests that concrete manipulatives fade away over time, it is important to remember that they fade away with one layer of abstraction and then reappear as a new layer is “piled” on.

3 Act Math Tasks: Curious Math iTunes U Course

Access over 30 tasks with all resources downloaded directly to your iOS Device to avoid streaming media in your classroom through the Curious Math iTunes U Course:

Enrol in Course

Hope you folks found this YCDSB math professional development experience useful.

I’d be delighted to come back and learn alongside you all again sometime soon!